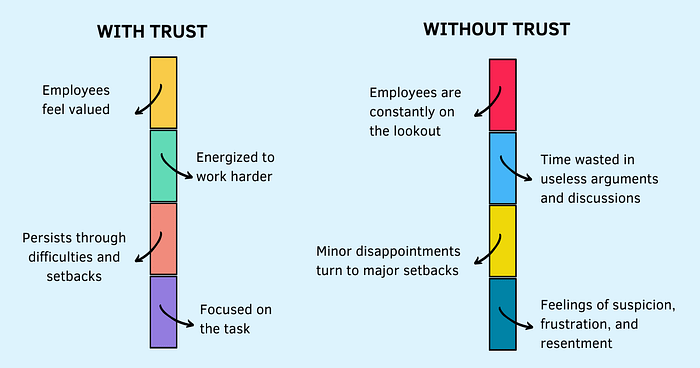
I am often surprised, if not shocked, when I see teams making big impact pricing decisions without calculating the variable costs to fix costs and the break-even point. Let’s walk through an example of a flower shop. The flower shop generates revenues of 450,000 and spends 216,000 on flowers, 150,000 on salaries, 60,000 on rent, and 50,000 on other costs. As a result, the annual loss is 26,000. On average, a customer spends $25 on a purchase and the shop counts 18,000 purchases per year. The holiday season and Valentine’s Day are of course the biggest sales driver. With this data, we can categorize the costs for flower as variable costs and all other expenses as fixed costs.

This means that if we sell more flowers, the costs for flowers go up, they are variable. But the salaries, the rent, and the other costs remained the same. They are fixed. We see that the average purchase generates revenues of $25 and direct costs of $12, which means that every purchase generates a gross contribution of $13. Put yourself in the shoes of the flower shop owner. What’s can you do to avoid another loss next year? 

Given the topic of this course, well, you can increase your prices, or alternatively, you can increase the volume, for example, by winning new clients. Of course, they are numerous other options, but let’s discuss price increase and volume increase. Before we increase our prices, we should answer one important question. At which price points are returning from losing money to generating a profit? In other words, at which price point is our profit exactly zero? 

This point is called break-even. You can calculate this break-even price by using a spreadsheet as shown here. You can use the what if function of your software that finds the price where profit zero. This price is $26.44, which is an increase of 5.78% compared to the starting price of $25. A more direct approach to calculating the break-even price starts with the total fixed costs, which are 260,000. If we sell 18,000 units, each unit must generate the gross contribution of $14.44 to cover the fixed costs. We then add $12 direct costs for the flowers we sell and end up with a break-even price of 26.44. The shop owner can also try to sell more flowers at the current price. The break-even question is, how many more flowers do they need to sell to cover all the costs? 

The direct calculation is similar as before. If every purchase generates a contribution of $13, they need to sell 2000 additional flower bouquets to cover the loss of $26,000. We can also use this spreadsheet to see that with 20,000 units sold, this is the initial 18,000 plus the additional 2000, the shop hits break-even. Please note that to eliminate the loss of $26,000, the owner can increase the price by 5.78% or increase the volume by 11%. This is a general rule. If you have to cover variable costs, a price increase is always more powerful than a volume increase. Let’s look at this further by increasing either the volume or the price by 10%. If the volume is increased by 10%, from 18,000 to 19,800, the loss is reduced from $26,000 to $2,600. However, if the price can be increased by 10%, from $25 to 27.50, the loss of $26,000 turns into a profit of $19,000. In any case (laughs) I strongly suggest that you buy more flowers for your loved one. 

It’s good for business and it’s good for you.